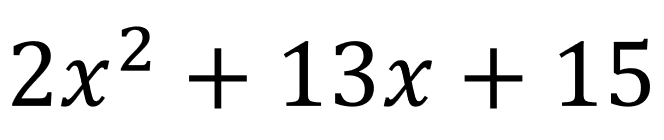Factor This! Breaking Down Quadratics with the Box Method
The Box Method: A Step-by-Step Guide to Factoring Quadratics
Factoring quadratics, or breaking down a polynomial into simpler parts, can seem confusing at first, but don't worry! There's a simple & easy-to-follow method called the box method that can help you get the hang of it. With this post, we are not going to dive too deep into the mathematics, but instead are going to learn a fun, simple way to turn quadratics into their factors using the box method. So regardless of your prior history with math, I hope you choose to take a look at the box method with me & enjoy learning some math today!
This is a polynomial. Specifically, it is a quadratic.
This is the same quadratic, but now split into its two factors on the right side of the equation. But how? Well, there are many ways to organize factoring quadratics like this.
One of my favorite ways to visually organize how to factor a quadratic like this is called the box method. Let’s walk through the steps to factor a quadratic with our example polynomial from earlier.
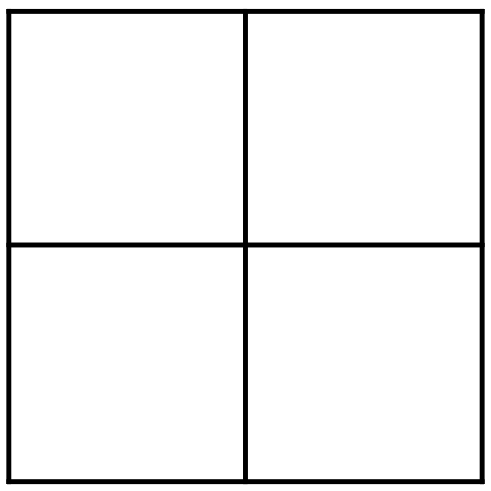
To get started, draw a large box. Inside that large box, draw four smaller boxes arranged in two rows & two columns.
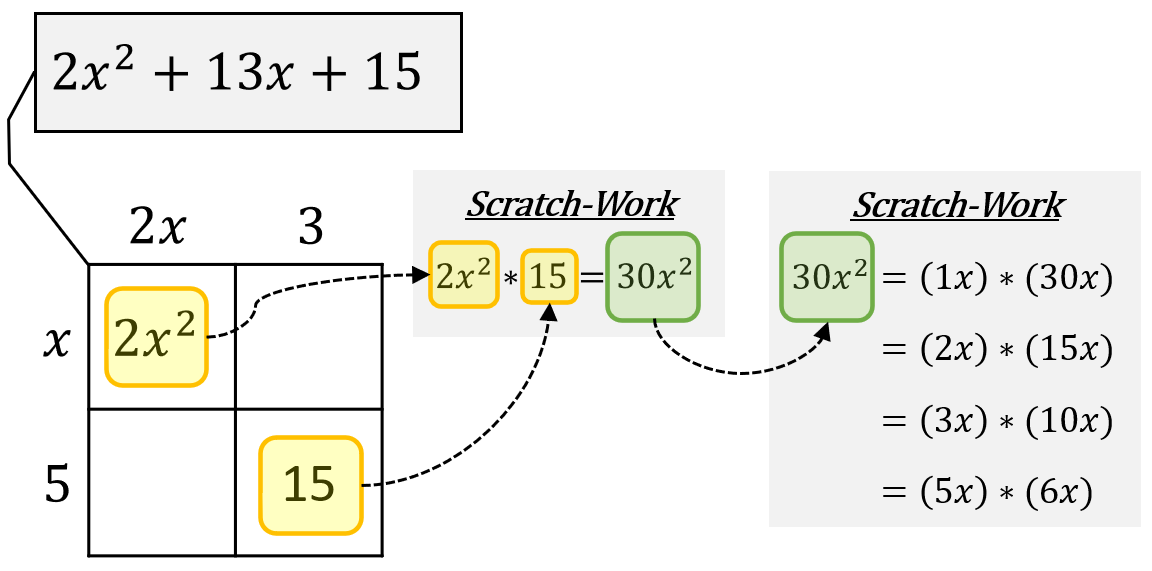
Next, put the 1st term (2x²) into the top-left box; & put the last term (15) into the bottom-right box.
Multiply the first term by the last term (2x²)(15) = 30x² & list out the factors of that product (30x²) looking for pairs which add up to the second term (13x). For example, (1x)(30x) is one way to get 30x², so we write that down in our Scratch-Work. (2x)(15x), (3x)(10x), & (5x)(6x) are the others.
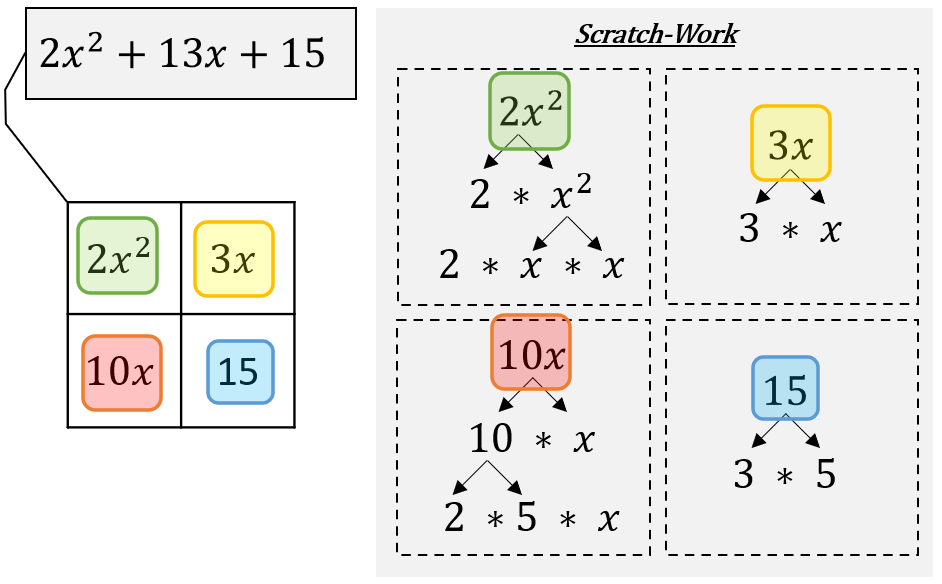
Since 3x & 10x add up to the second term (13x), we put 3x & 10x in the top-right & bottom-left boxes. Note - it does not matter which goes bottom-right or which goes top-left here.
Next, we need to break each of our four pieces into their simplest factors using factor trees. (Want to learn more about factor trees & how they can be used when factoring monomials like these? Check out my other blog post here: Coming Soon)
Now find the Greatest Common Factor (GCF) of each row pair & write them down in that row on the left side of the box; & find the Greatest Common Factor (GCF) of each column pair & write them down in that column on the top side of the box.
I’ve done one pair for you - for the left column, the Greatest Common Facor of 15 & 10x is 5, and we look at the bottom-left box to determine the sign. Because 10x is positive, we write positive 5 on the left side of that row of our box.
Take a moment to confirm you understand how to determine the other three pairs.
For the right column, the Greatest Common Factor of 2x² & 10x is 2x and it re. Can you see why by observing the factor tree?
The numbers you wrote on the top of the box are the pieces that form one factor (2x+3) & those you wrote on the left side of the box are the pieces that form the other factor (x+5).
& just like that, we have successfully used the box method to factor a quadratic. The box method helps you to organize the problem at hand & keep track of all of the parts as you factor. By using the box method, you are empowered with the ability to factor quadratics without losing any terms! Remember, the aim of this journey was not to find the perfect answers, but to foster curiosity & the desire to discover; so don't be afraid to think differently & challenge yourself. Until next time, keep exploring & keep learning!
Math-y Note: Two things. Prior to factoring using the box method:
Make sure you factor the polynomial until the greatest common factor of the coefficients is equal to 1.
If the highest power term’s coefficient is negative, factor a (-1) out of the polynomial.
Want more practice factoring quadratics with the box method? Check out My TPT.com Resource: (Coming Soon).
Want more box method but in the opposite way? (What does that even mean?) Check out Box It Up: The Simple Solution to Multiplying Polynomials.
Further Questions to Ponder:
Does using the box method like this ever not work? How can you figure that out?
Does this method work for different types of polynomials, such as cubic? x³+2x²-5x-10 for example?
What other ways can factoring quadratics be visualized? (Stay tuned to Mister Marx’s Math Corner for more!)
This post is part of Mister Marx’s Power Pair Series of blog posts. Factor This! Breaking Down Quadratics with the Box Method pairs with Box It Up: The Simple Solution to Multiplying Polynomials. Together, the pair of blog posts highlight the various ways the box method can be used.