The Power of Pascal’s Triangle: Exploring Mathematical Patterns
Hello, seekers of knowledge! Are you ready to explore one of my favorite sources of mathematical patterns: Pascal's Triangle? This mathematical construct, which might appear simple at first glance, holds deep significance and finds wide application in areas such as combinatorics, algebra, and calculus. Don't fret, you don't need any specialized math skills to participate in this adventure. Today, we will be solely focusing on recognizing patterns both simple & complex within Pascal’s Triangle.
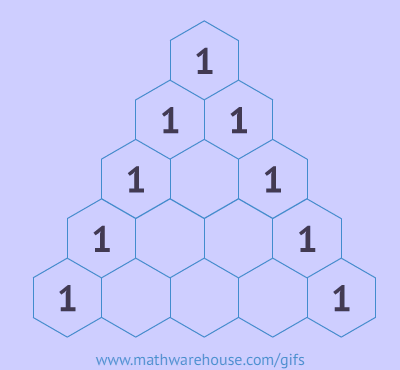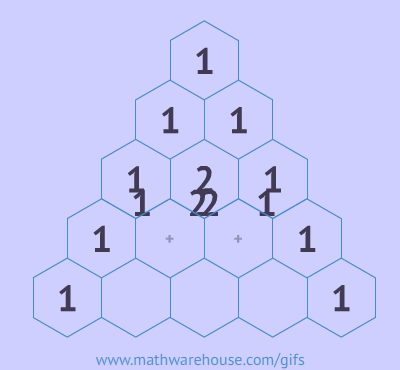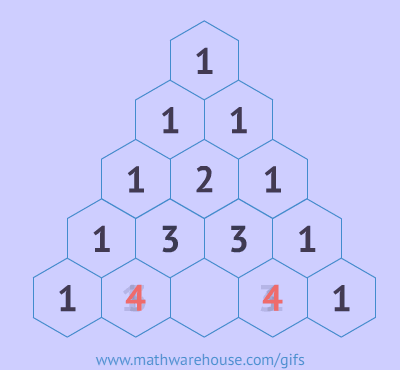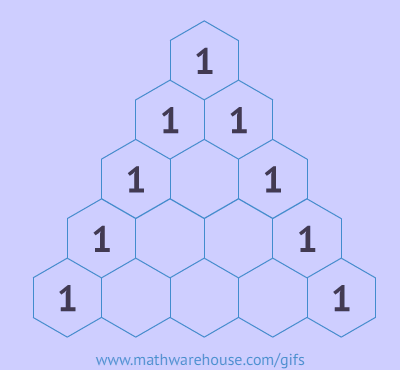
Take a look at this animated gif. It showcases numbers being added in a triangle pattern, creating what's known as Pascal's Triangle. Have you ever thought that such a simple procedure can reveal innumerable, profound mathematical relationships? It's an intriguing notion, and one that I urge you to explore.
As you delve into this gif, notice how each number is calculated within the triangle, contributing to the formation of Pascal's Triangle. Here are some questions to pique your curiosity:
How would you describe how the RED numbers are being created in Pascal’s Triangle?
What patterns do you see across the diagonals of Pascal’s Triangle?
What patterns do you see across the rows of Pascal’s Triangle?
What other patterns do you see in Pascal’s Triangle?
With your exploration started, I encourage you to grab a pencil & paper and try building Pascal’s Triangle yourself! How many rows can you build? How many patterns can you find? There are countless patterns to be discovered - have fun!
Remember, exploration and discovery are the bedrocks of true learning. Don't hesitate to ponder, be curious, and draw connections. And most importantly, applaud yourself for taking the time to uncover a new nugget of knowledge today! Enjoy your expedition!
BONUS: Ever heard of the Binomial Theorem? Keep your eyes peeled for an upcoming blog post on the Binomial Theorem to learn more & see how Pascal’s Triangle originates from the coefficients in the Binomial Theorem!
BONUS 2: Ever heard of the Fibonacci Sequence? Keep your eyes peeled for an upcoming blog post on the Fibonacci Sequence to learn more & see how the Fibonacci Sequence is hidden within the patterns of Pascal’s Triangle!