Comment Chronicles: Multiplying Polynomials With The Carve Copy Distribute (CCD) Method
Hello! Today, I have something special to share with you. In a recent post, one of our valued community members introduced me to a fantastic new approach to polynomial multiplication. I couldn't be more excited to share this with you & give them the recognition they deserve! Enjoy the first post in my new series: Comment Chronicles where I debut a newly-created #MathGIF inspired by our community.
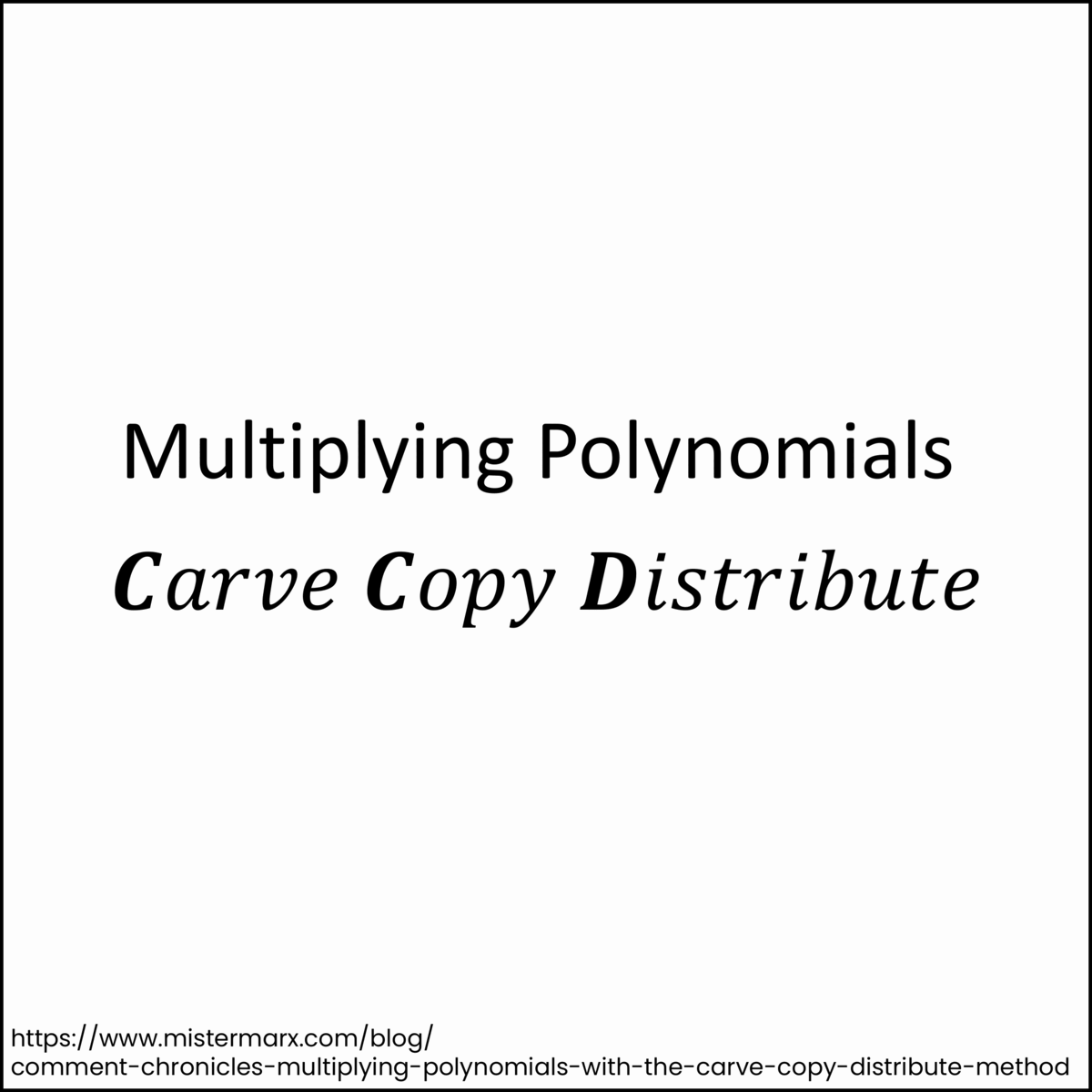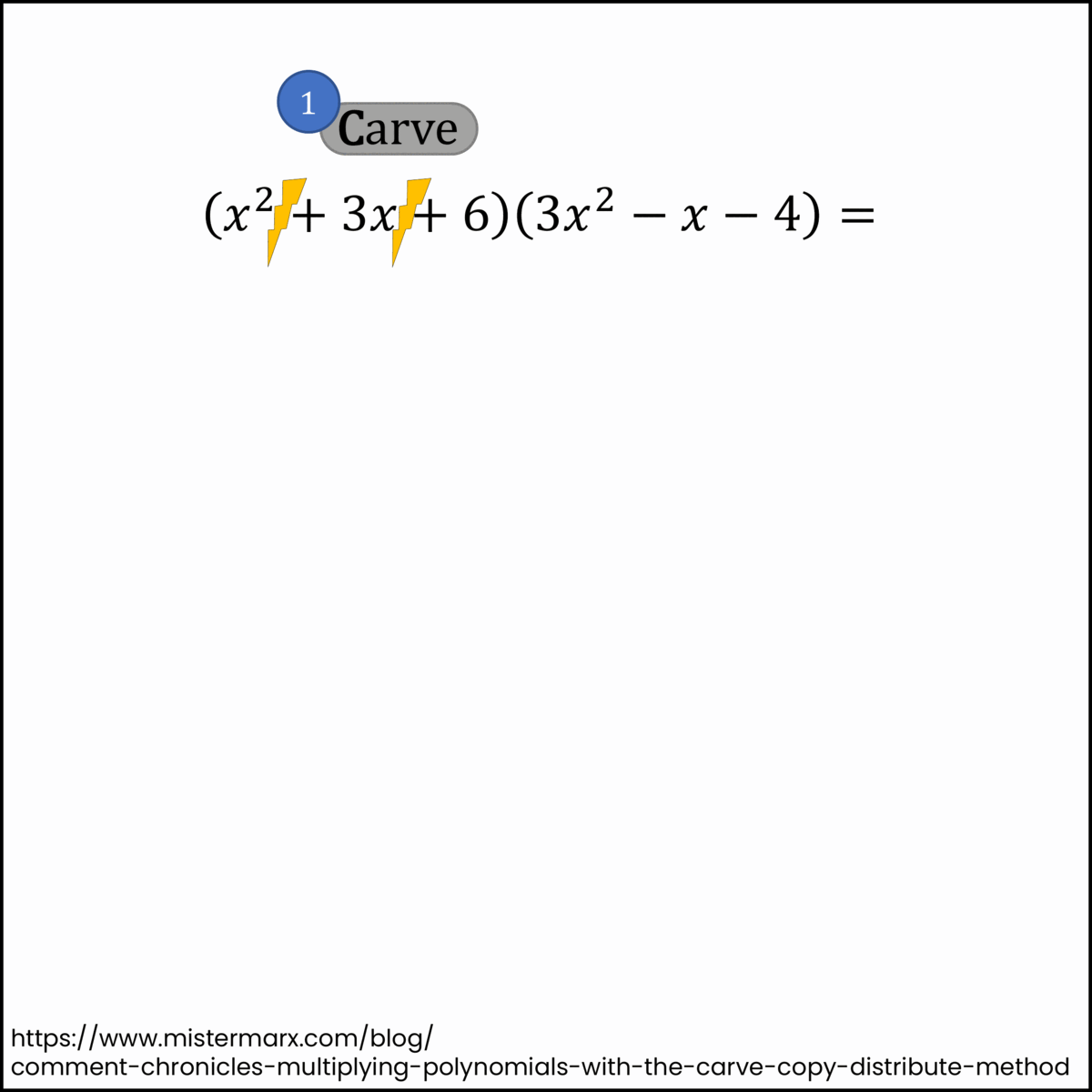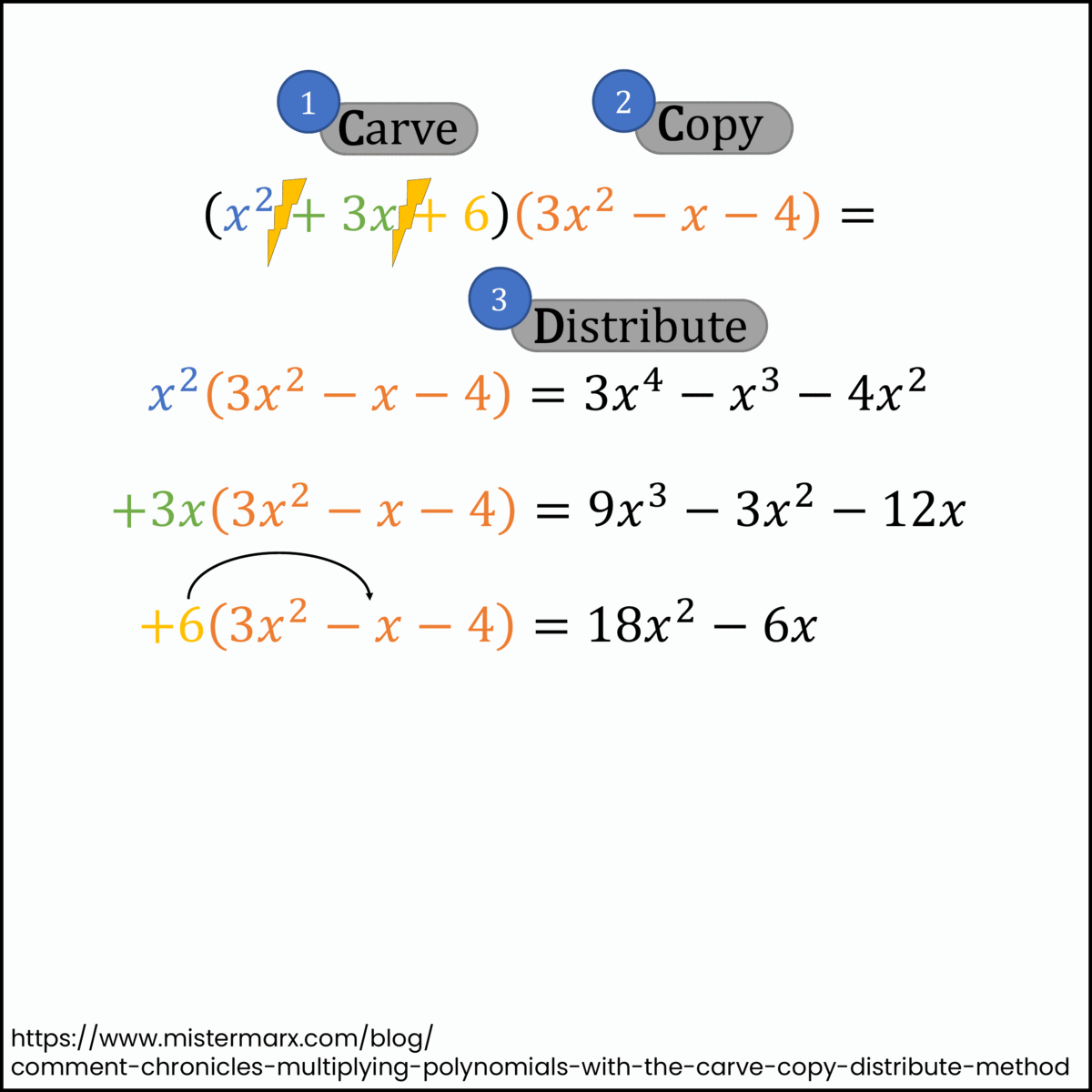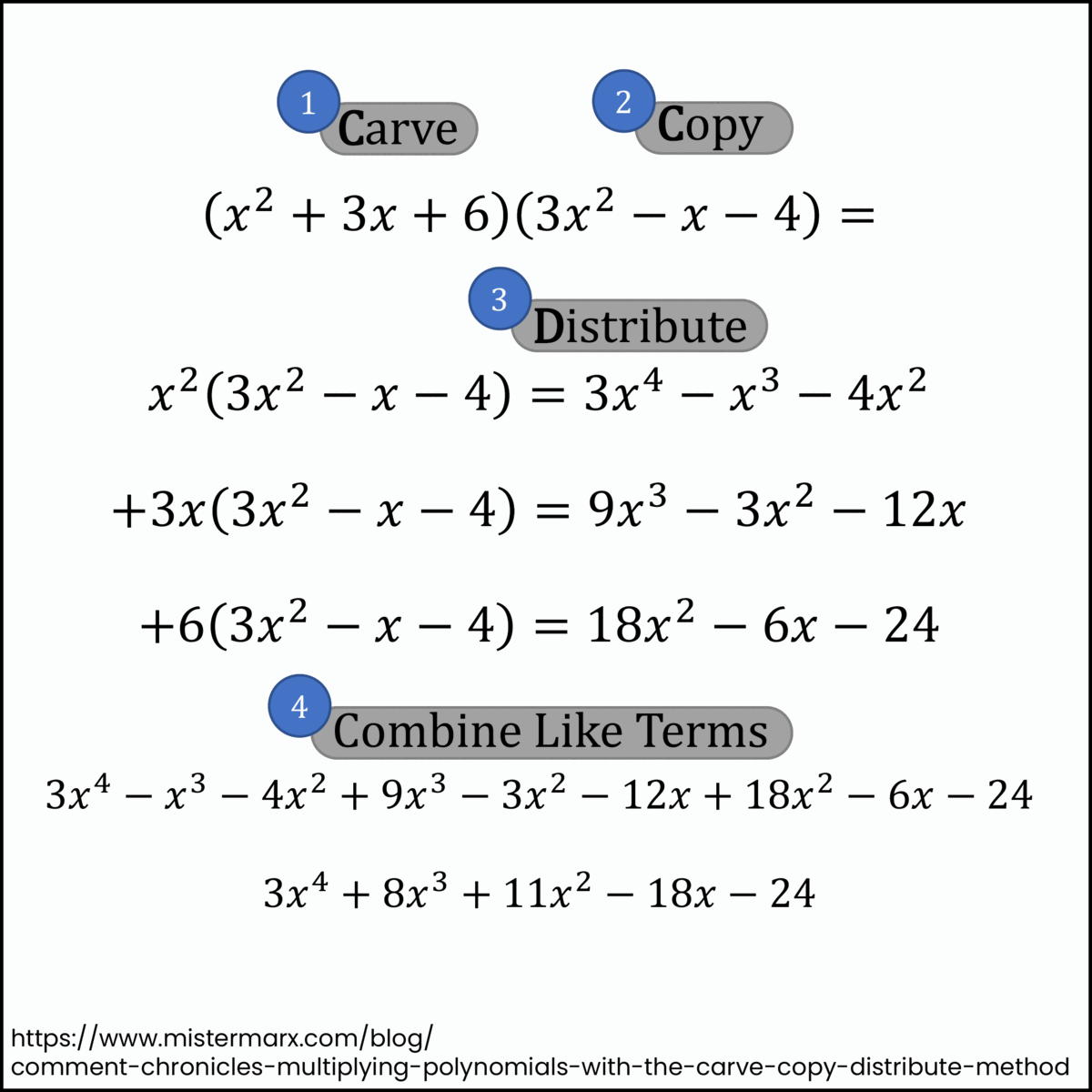
In a recent comment, this commenter introduced us to the Carve Copy Distribute (CCD) Method for polynomial multiplication, an alternative to the well-known FOIL Method, Area Model, & Box Method for multiplying binomials. I always strive to provide students with gateways to entry that meet them where they are & equip them with as many tools as possible moving forward. This CCD Method is a perfect example of a scalable technique that can be applied to multiplying polynomials of all degrees.
Upon learning about the Carve Copy Distribute Method, I couldn't help but appreciate its versatility. Unlike the FOIL Method -which is limited to binomial multiplication - the CCD Method can be used for multiplying polynomials of any degree. This makes it an incredibly valuable resource for students, as it can be used throughout their evolving mathematical learning journey.
This fantastic method is a testament to the power of our community, & I am thrilled to be able to share it with all of you. You can see the Carve Copy Distribute Method in action in the #MathGIF below. As you watch it, I encourage you to share what you notice & what you wonder about the animated gif in the comments below.
As you continue to observe this gif, consider the following questions:
Does using the Carve Copy Distribute Method like this ever not work? How can you figure that out?
Does this method work for all different types of polynomials, such as those with more than four terms? (2x³+3x²-4x+1)(x²-2) for example?
Again, massive shoutout & thank you to <Julie Auxier (Balabon) via Facebook> for introducing me to this new approach! I am eager to see what other innovative ideas our community has in store. Don't hesitate to share your thoughts in the comments section below.
Happy math’ing everyone!
Once again, thank you <Julie Auxier (Balabon) via Facebook> for sharing your method!
